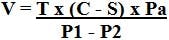Optimize compressed air storage to drive system-wide energy efficiency

Where:
V = Receiver volume required, ft3
T = Time allowed for pressure drop, minutes
C = Intermittent demand, acfm
S = Compressor capacity minus process demand, acfm
Pa = Absolute atmospheric pressure, psia
P1 = Initial receiver pressure, psig
P2 = final receiver pressure, psig

In the past, the recommended storage receiver size was 1 gallon per each cubic foot per minute (cfm) of the system air compressor capacity. For example, if the compressor was a 100 hp, 400 cfm rated unit, then a storage receiver sized at about 400 gallons was used. This sizing guideline was common when most lubricated screw air compressors were running in inefficient modulation mode pressure control. (Modulation control is where the compressor inlet valve is progressively closed off by the local control system to control the output flow. In modulation mode, the power consumption only reduces 3 percent for each 10 percent reduction in flow.)

Over the years, load/unload control mode has become the most common control method because it is a more efficient way to run lubricated screw compressors than modulation mode at loads that are lower than the full compressor capacity. Load/unload controlled compressors cycle between loaded and unloaded condition at part loads. In load/unload control mode, the theoretical ideal power turn down is about 7 percent for each 10 percent reduction in flow. But with lubricated screw compressors, this turn down is affected by load/unload cycle frequency.

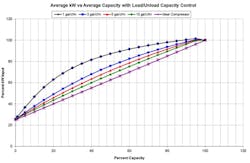
The characteristic cycle frequency of a load/unload compressor depends on the width of the compressor pressure band, the volume of the effective system storage, and the loading percentage of the compressor. The higher the cycle frequency, the less efficient the compressor runs at part loads, especially if it is a lubricant injected compressor. The changes to the power vs. flow characteristics with changing storage receiver sizes are shown in Figure 1.

The load/unload state of the compressor is controlled by the setting in compressor pressure controller, usually set with a band which is 10 psi wide. If the old "1 gallon per cfm" rule is applied to a compressor with a 10 psi wide setting, the expected theoretical efficiency gain due to switching from modulation to load/unload control would not materialize because the load/unload cycle frequency is too high, as seen on the top dark blue curve in Figure 1. This problem has led compressor suppliers to upgrade the industry rule of thumb to "2 gallons per cfm". Increasing the capacity to this level reduces the cycle frequency by a factor of 2 but the compressors still have marginal efficiency at some part loads.

In examining Figure 1, if a compressor with 100 kW full load power and 1 gallon per cfm of storage capacity was operating at 40% load, it would consume about 82 kW. If that same compressor was working with 3 gallons per cfm of storage, the power consumption would reduce to about 68 kW for energy savings of 17%. This is still significantly different than the expected 55 kW power consumption for 33% savings if the ideal compressor curve is used to calculate the expected savings.

{pb}

Storage capacity and pressure stability

[sidebar id="7"]

Before discussing the improvement of pressure stability that goes hand-in-hand with larger storage capacity, let's go through a storage capacity primer. Let’s say you have purchased a large storage tank and it is sitting unpressurized out on the pad. How much air does it contain? If it is a 1,060 gallon receiver it will contain 1,060 gallons of atmospheric air pressurized to the local atmospheric pressure (14.5 psi above absolute zero for example). Since every 7.48 gallons contains one cubic foot, that receiver contains about 142 cubic feet (cf) of ambient air.

If one more atmosphere of air pressure is added to the receiver–assumed here to be 14.5 psi absolute–or another 142 cf, the pressure in the receiver will rise by 14.5 psi. This continues as more air is added to the receiver with each 142 cf (1,060 gallons) adding another 14.5 psi.

If you want to determine how much air to add in order to boost the pressure in the tank by 1 psi, simply divide 142 cf by 14.5 psi, with the result yielding 9.78 cf/psi. This number is important, because it indicates how much the pressure will change in the tank if a certain amount of compressed air is added or removed from the storage tank.

For example, if a compressed air powered machine uses 97.8 cf more than the compressor could produce, this air must be supplied by the receiver. For the tank in question, the pressure in the storage tank would drop 97.8 cf divided by the 9.78 cf/psi capacity of the storage receiver (i.e., 10 psi) during that event if all the air came from the tank storage. If the tank size was doubled, then the pressure would drop only 5 psi, and if tripled only 3 psi, and so on. This shows that if we desire pressure stability, and the compressed air system has high transient demands, then the way to protect production machinery from low pressure is often to add more storage.

Taking another example that illustrates this point, suppose we have a 100 hp (85 kW) compressor that is rated at 400 cfm operating in a system with 400 gallons (3.7 cf/psi) of storage capacity, sized using the 1 gallon per cfm rule of thumb. Perhaps the production machinery in this system starts to have problems if the system pressure drops below 90 psi. Let’s also assume that the compressor is operating at an unload pressure 110 psi and a load pressure of 100 psi.

If a transient one-minute 500 cfm demand event hits the system, the capacity of the compressor will be exceeded by 100 cfm and air will be consumed from the storage receiver while the system pressure drops. A worst-case scenario would be to have this demand hit the system when the system pressure is right at the 100 psi load point. If this was the case, the pressure would fall by 100 cf (the shortfall) divided by 3.7 cf/psi, or 27 psi per minute. At this rate it would take only about 20 seconds for the pressure to fall below the 90 psi pressure required by the production machinery. But if the storage capacity is increased to 5 gallons per cfm (2,000 gallons at 18.4 cf/psi) the pressure during this worst-case scenario would only fall by 5.4 psi during the one-minute event.

For these conditions, the old "1 gallon per cfm" storage capacity rule, or even 2 gallons per cfm, would not be adequate. The only alternative would be to run another compressor to supply the additional 100 cfm. If the other compressor was another 100 hp compressor, we can see by the curve in Figure 1 that it would cost about 70% of its full load power to run at 25% capacity (or about 60 kW), compared to no power consumed by a large storage tank. After the one-minute event the compressor would typically run at 25% power at zero flow for a cool down period before it could shut off, wasting even more power.

{pb}

Storage to protect against equipment failure

Another condition that might require more storage than the rule of thumb is when designing to protect the system against equipment failure. Using the second example above, if the 400 cfm compressor tripped off while running fully loaded, this would remove 400 cf per minute of compressor capacity from the system. If the 2,000 gallon system experienced this problem when the compressor was running at full load with the transient demand, the pressure would start to drop by 500 cfm divided by 18.4 cf/psi, or 27 psi per minute. If it takes 30 seconds to automatically start and load a backup compressor, and the backup is set to start at 95 psi, the pressure will have dropped 13.5 psi to 81.5 psi by the time the backup compressor saves the pressure, which is lower than our 90 psi minimum pressure requirement.

[sidebar id="7"]

If we wanted to ensure adequate pressure at all times, even with main compressor failure, then at least 5,420 gallons of storage (50.0 cf/psi) would need to be installed. The "2 gallons per cfm" rule would be inadequate, as the required size to protect this system is the equivalent to at least 13.6 gallons per cfm compressor capacity.

An alternative to this would be to jack the system pressure up by 10 psi to give the system more time for the spare compressor to start. However, for every 2 psi increase in pressure the loaded compressor consumes about 1% more power, reducing system efficiency for all operating hours.

Ron Marshall is a certified engineering technologist in the province of Manitoba and has received certification as an energy manager, demand side management and measurement and verification professional through the Association of Energy Engineers. He was the first Canadian participant to qualify as a DOE AIRMaster+ specialist and is involved as a member of Compressed Air Challenge's Project Development and Marketing Committee. Marshall has worked in the industrial compressed air field for 16 years as an industrial systems officer for Manitoba Hydro's Customer Engineering Services Department, and he is Manitoba Hydro's industrial compressed air systems expert whose efforts support the utility's Power Smart Performance Optimization program. Contact him at [email protected].